An eikonal based formulation for traveltime perturbation with respect to the source location

Lens example

Since the differential equation depends on velocity changes in the direction of the source shift, we test the methodology on a model that contains a lens anomaly in an otherwise constant velocity gradient ( $\frac{dv}{dx}=0.5 s^{-1}$ and $\frac{dv}{dz}=0.7 s^{-1}$ with velocity at the origin equal to 2 km/s) model. The lens is located at 600 meters laterally and 500 meters depth with a velocity perturbation of +500 m/s (or 20%). The lens has a diameter of 200 meters and causes a large velocity variation. Using this model, we test the accuracy of the first-order, second-order, and the Shanks-transform representation equations.

For a source located at 200 meters lateral distance from origin and 200 meter depth, we solve the eikonal equation using the fast marching method with second order accuracy. The traveltime field in this case is represented by the solid contours on the left side plots of Figures 4, 5, and  6. We also solve the eikonal equation for source located virtually 100 meters away in lateral direction and it is represented by the solid curves in the middle plot of the three Figures. Solving for $D_x$ using equation 3 and using that along with the original traveltime field, we obtain an approximate traveltime field for a source 100 meters away. This new traveltime field is represented by the dashed contour curves in Figure 4. The absolute difference between the simulated traveltime and the true one both displayed in the center plot is given by the density plot shown on the right side of Figure 4.

circ1
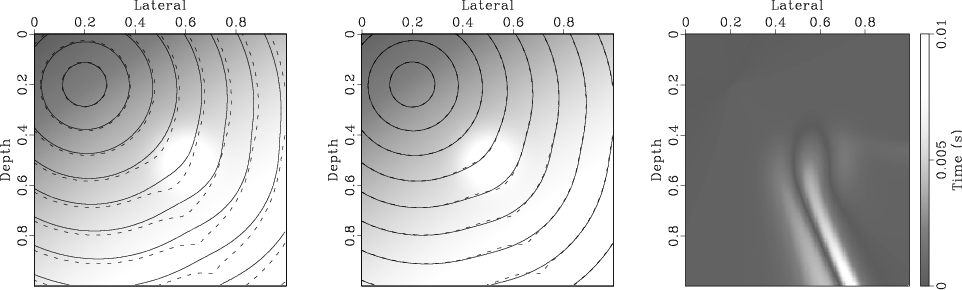
Figure 4. The traveltime contour (solid curve) plot for a source at lateral and depth position of 0.2 km (left) and for a source virtually perturbed by 100 meters in the lateral direction (middle), both compared with the traveltime derived using the first-order accuracy perturbation eikonal for a 100 meters virtual shift (dashed curves). In both plots the velocity field is shown in the background. Also shown on the right is a density plot of the difference between the two contours in the middle plot.

The errors are generally small (less than 0.008 s), with the largest of errors appearing on the lower side of the lens. This error is generally small considering the large shift (100 meters) and first-order nature of the expansion. In addition, errors for the rest of the traveltime field corresponding to the linear variation in velocity is extremely small.

circ2
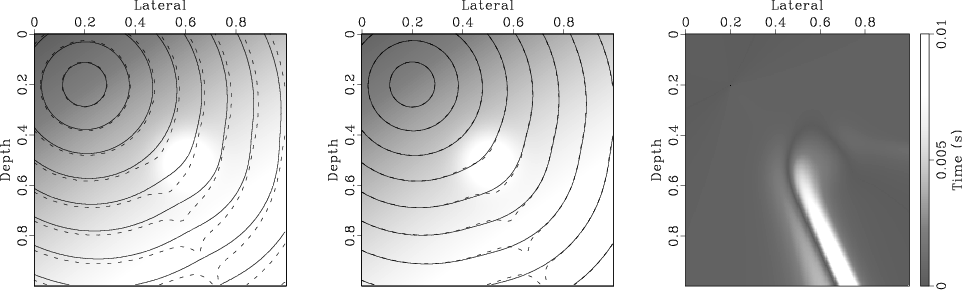
Figure 5. The traveltime contour (solid curve) plot for the original source (left) and for a source virtually perturbed by 100 meters in the lateral (middle), both compared with the traveltime derived using the second-order accuracy perturbation eikonal for a 100 meters virtual shift (dashed curves). In both plots the velocity field is shown in the background. Also shown on the right is a density plot of the difference between the two contours in the middle plot.

Figure 5 is similar to Figure 4, but now we use the second-order expansion, which requires solving the linear partial differential equation twice. Overall, as expected, the errors are less than the first order case with clear reduction in the upper side trail of the lens.

circ3
Figure 6. The traveltime contour (solid curve) plot for the original source (left) and for a source virtually perturbed by 100 meters (middle), both compared with the traveltime derived using Shanks transform perturbation eikonal for a 100 meters virtual shift (dashed curves). In both plots the velocity field is shown in the background. Also shown on the right is a density plot of the difference between the two contours in the middle plot.

With hardly any additional computational cost, we can use the Shank transform representation of the expansion and in this case the errors, as shown in Figure 6, are reduced even further.

circ4
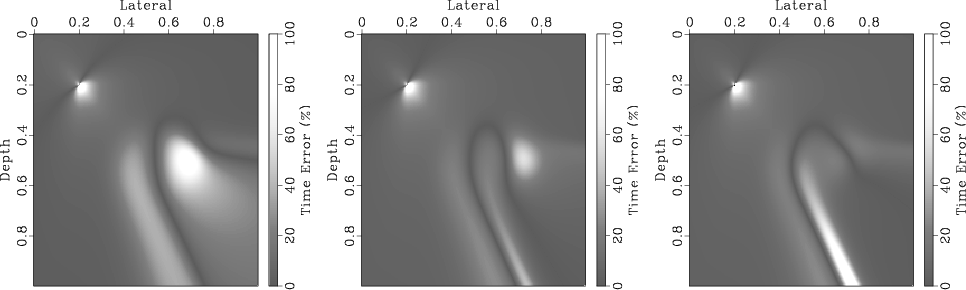
Figure 7. A density plot of the traveltime error in percent for the difference plots in Figures 4-6 (right), plotted from left to right, respectively. The percent error is measured in a relative manner where 0 corresponds to the accurate traveltime and 100% to the unperturbed traveltime.

To emphasize the role of the perturbation terms in approximating the source-shifted traveltime, we define a relative percent error as

$$t_{err} = 100 \frac{\tau - \tau_0}{\tau_1 - \tau_0},$$ (24)

where $\tau_0$ is the unperturbed traveltime for the original source, $\tau_1$ is the traveltime for the desired source calculated directly using the conventional eikonal equation, and $\tau$ is the traveltime estimated using the perturbation equations for the desired source. If $\tau$ is equal to $\tau_1$, as desired, the error is zero. However, if $\tau$ equals the unperturbed traveltime $\tau_0$ the error is 100 percent. Figure 7 shows this relative errors for the first-order accuracy perturbation (left), the second-order accuracy perturbation (middle), and using Shanks transform perturbation (right) for the linear model with a lens. The errors are overall less for the Shanks transform perturbation. The large error at position and depth equal to 0.2 km corresponds to the source location, where the denominator of equation 24 tends to zero.

An eikonal based formulation for traveltime perturbation with respect to the source location