Fast algorithms for elastic-wave-mode separation and vector decomposition using low-rank approximation for anisotropic media

A 3D two-layer TI model

We also test the mode separation approach on a 3D two-layer TI model, with $v_{p0}=2500 m/s$ , $v_{s0}=1200 m/s$ , $\epsilon=0.25$ , $\delta=-0.25$ and $\gamma=0$ in the first layer, and $v_{p0}=3600 m/s$ , $v_{s0}=1800 m/s$ , $\epsilon=0.2$ , $\delta=0.1$ and $\gamma=0.05$ in the second layer. The size of the model is $N_{x}=201\times201\times201$ . A displacement source located at the center of the model and oriented at tilt $45^{\circ}$ and azimuth $45^{\circ}$ . Figure 5 displays the elastic wavefields and the separated qP-, qSV- and SH-wave fields using the low-rank approximate algorithm. Because the substantial increase of the model size, it is still time consuming to separate the 3D wave modes even if the proposed fast algorithm is used. It took 4008.0, 4130.0 and 91.8 seconds to construct the separated forms of the mode separation matrixes for qP-, qSV- and SH-waves, respectively. For one time step, it took 61.4 seconds to extrapolate the elastic wavefield, and 15.2, 15.8 and 6.8 seconds to separate qP-, qSV- and SH-wave fields with the rank $N=M=2$ .

For comparison, we only change the second layer to a TTI medium with a tilt angle $\theta=30^{\circ}$ and azimuth $\phi=30^{\circ}$ (other paramters continue to use). Figure 6 displays the corresponding elastic wavefields and their mode separation results. It took 4087.8, 4280.8 and 206.2 seconds to construct the separated forms of the mode separation matrixes for qP-, qSV- and SH-waves, respectively. For one time step, it took 101.0 seconds to extrapolate the elastic wavefield, and 15.2 and 15.8 seconds to separate qP- and qSV-wave modes with the rank $N=M=2$ . It took 14.1 seconds to separate SH-wave with the rank $N=M=2$ . As we observed, the most time-consuming task here is to construct the separated forms of the mode separation matrixes. More CPU time is required to separate SH-wave in 3D TTI media as well.

Polxp1,Polzp1,Polxp2,Polzp2,Errpolxp1,Errpolzp1,Errpolxp2,Errpolzp2
Figure 1. Low-rank approximate mode separators of qP-wave in a 2D two-layer TI model: (a) $a_{px}(\mathbf {x},\mathbf {k})$ and (b) $a_{pz}(\mathbf {x},\mathbf {k})$ constructed by using low-rank decomposition in the VTI layer; (c) $a_{px}(\mathbf {x},\mathbf {k})$ and (d) $a_{pz}(\mathbf {x},\mathbf {k})$ constructed by using low-rank decomposition in the TTI layer; (e), (f), (g) and (h) represent the low-rank approximation errors of these operators. According to the qP-qSV mode polarization orthogonality, we have the following relations: $a_{svx}(\mathbf {x},\mathbf {k})=-a_{pz}(\mathbf {x},\mathbf {k})$ and $a_{svz}(\mathbf {x},\mathbf {k})=a_{px}(\mathbf {x},\mathbf {k})$ . Therefore, the above pictures also demonstrate the low-rank approximate separators and their errors for qSV-wave.

ElasticxInterf,ElasticzInterf,ElasticSepPInterf,ElasticSepSVInterf
Figure 2. Elastic wave mode separation in the two-layer TI model: (a) x- and (b) z-components of the synthetic elastic displacement wavefields synthesized at 0.3s; (c) and (d) are the separated scalar qP- and qSV-wave fields using low-rank approximation.

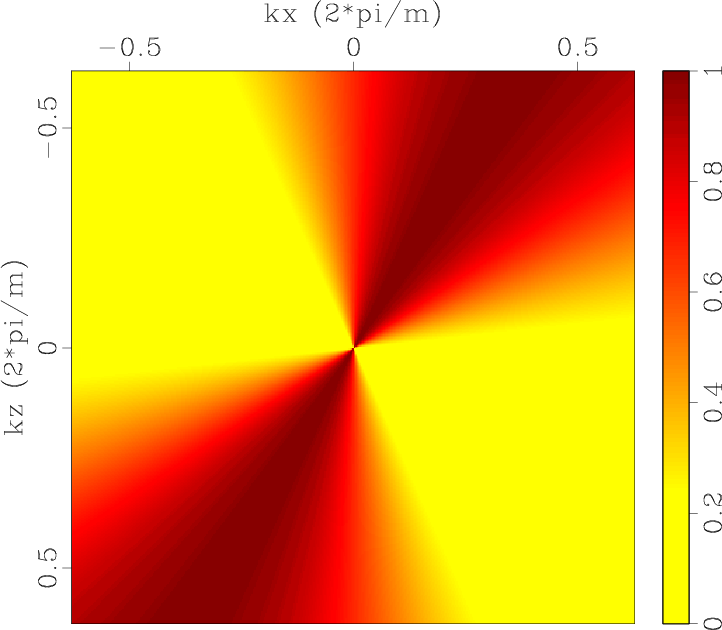
Decompxp1,Decompzp1,Decompxzp1,Errdecxp1,Errdeczp1,Errdecxzp1,Decompxp2,Decompzp2,Decompxzp2,Errdecxp2,Errdeczp2,Errdecxzp2
Figure 3. Low-rank approximate vector decomposition operators of qP-wave in the 2D two-layer TI model: (a) $a_{px}(\mathbf {x},\mathbf {k})a_{px}(\mathbf {x},\mathbf {k})$ , (b) $a_{pz}(\mathbf {x},\mathbf {k})a_{pz}(\mathbf {x},\mathbf {k})$ , and (c) $a_{px}(\mathbf {x},\mathbf {k})a_{pz}(\mathbf {x},\mathbf {k})$ , and (e), (f) and (g) represent their low-rank approximation errors in the VTI layer. (h), (i), (j), (k), (l) and (m) are these operators and their low-rank approximation errors in the TTI layer.

ElasticPxInterf,ElasticPzInterf,ElasticSVxInterf,ElasticSVzInterf
Figure 4. Elastic wave vector decomposition in the two-layer VTI/VTI model: (a) x- and (b) z-components of vector qP-wave fields; (c) x- and (d) z-components of vector qSV-wave fields.

ElasticxInterf,ElasticyInterf,ElasticzInterf,ElasticPInterf,ElasticSVInterf,ElasticSHInterf
Figure 5. Elastic wave mode separation in the 3D two-layer VTI model: (a) x-, (b) y- and (c) z-components of the synthetic elastic displacement wavefields synthesized at 0.17s; (d) qP-, (e) qSV- and (e) SH-wave fields separated from the elastic wavefields.

ElasticxInterf,ElasticyInterf,ElasticzInterf,ElasticPInterf,ElasticSVInterf,ElasticSHInterf
Figure 6. Elastic wave mode separation in the 3D two-layer VTI/TTI model: (a) x-, (b) y- and (c) z-components of the synthetic elastic displacement wavefields synthesized at 0.17s; (d) qP-, (e) qSV- and (e) SH-wave fields separated from the elastic wavefields.

Fast algorithms for elastic-wave-mode separation and vector decomposition using low-rank approximation for anisotropic media