|
|
|
|
On anelliptic approximations for qP velocities in TI and orthorhombic media |
Previous researchers have shown that in TI and orthorhombic media, only three and six combinations of stiffness coefficients respectively are sufficient to describe qP-wave propagation. The most widely used parameters are Thomsen's parameters (Thomsen, 1986) in TI media and their extension to orthorhombic media (Tsvankin, 1997). Another notable parameterization scheme involves the ![]() parameter (Alkhalifah and Tsvankin, 1995) and is commonly used when the so-called acoustic approximation is assumed (Alkhalifah, 1998,2000b,2003,2000a). In this study, following the work of Muir and Dellinger (1985) and Dellinger et al. (1993), we adopt the set of anisotropic parameters, referred to as Muir-Dellinger parameters, which represent different combinations of elastic moduli.
parameter (Alkhalifah and Tsvankin, 1995) and is commonly used when the so-called acoustic approximation is assumed (Alkhalifah, 1998,2000b,2003,2000a). In this study, following the work of Muir and Dellinger (1985) and Dellinger et al. (1993), we adopt the set of anisotropic parameters, referred to as Muir-Dellinger parameters, which represent different combinations of elastic moduli.
In our notation, the Muir-Dellinger parameters include ![]() ,
, ![]() ,
, ![]() , and
, and ![]() , where
, where ![]() denotes the horizontal velocity squared and
denotes the horizontal velocity squared and ![]() denotes the vertical velocity squared, and
denotes the vertical velocity squared, and ![]() and
and ![]() are anelliptic parameters derived from fitting the phase velocity curvatures along the horizontal axis and vertical axis. In terms of density-normalized stiffness tensor coefficients in Voigt notation,
are anelliptic parameters derived from fitting the phase velocity curvatures along the horizontal axis and vertical axis. In terms of density-normalized stiffness tensor coefficients in Voigt notation,
![]() ,
,
![]() , and
, and
| Anelliptic | Thomsen |
|
|
|
|
|
|
| Schemes | Parameters | Elliptical Ani. | Acoustic Approx. |
| Thomsen (1986) |
|
||
| Alkhalifah (1998) | |||
| Muir and Dellinger (1985), | |||
| Fomel (2004), and proposed | |||
To study correlations between anisotropic parameters, we compiled laboratory measurements of stiffness tensor elements or other equivalent measurements of anisotropy (Jones and Wang, 1981; Thomsen, 1986; Vernik and Liu, 1997; Wang, 2002; Schoenberg and Helbig, 1997). Computing ![]() and
and ![]() according to equations 1 and 2, we discover an empirical relationship between anelliptic parameters shown in Figure 1. The results suggest that
according to equations 1 and 2, we discover an empirical relationship between anelliptic parameters shown in Figure 1. The results suggest that ![]() and
and ![]() exhibit a linear relationship (
exhibit a linear relationship (
![]() ) that appears to depend solely on lithology, regardless of the geographical location of the samples. We can also observe that the proportionality constant
) that appears to depend solely on lithology, regardless of the geographical location of the samples. We can also observe that the proportionality constant ![]() is close to 1 for nearly isotropic rocks (carbonates and sandstones) but deviates from 1 for highly anisotropic rocks (shales). We assume that each relationship between
is close to 1 for nearly isotropic rocks (carbonates and sandstones) but deviates from 1 for highly anisotropic rocks (shales). We assume that each relationship between ![]() and
and ![]() given in Figure 1 is valid for that type of media and therefore, the proportionality constant
given in Figure 1 is valid for that type of media and therefore, the proportionality constant ![]() and the intercept
and the intercept ![]() are not new independent parameters.
are not new independent parameters.
In consideration of a linear relationship (
![]() ) above, the ratio of veritical qP- and qSV-wave velocites squared
) above, the ratio of veritical qP- and qSV-wave velocites squared
![]() can be expressed as,
can be expressed as,
![$\displaystyle R = \frac{(1+2\epsilon)[(1+2\delta)(a-1) + b(1+2\epsilon)]}{a(1+2\delta)+(1+2\epsilon)(b-1-2(\delta-\epsilon))}~.$](img44.png) |
(3) |
| (4) |



|
|---|
|
qshalelegnew,qsandlegnew,qcarbonateleg
Figure 1. Relationship between |
|
|
Because the in-plane qP-wave propagation in orthorhombic media behaves identically to the case of TI media (Tsvankin, 2012), we can extend the Muir-Dellinger parameters from 2D to 3D appropriately for studies of orthorhombic media. The full set of parameters in 3D includes ![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() , and
, and ![]() , where
, where ![]() denotes the velocity squared in the
denotes the velocity squared in the ![]() direction and
direction and ![]() denotes the anelliptic parameters derived from fitting the phase velocity curvatures along the
denotes the anelliptic parameters derived from fitting the phase velocity curvatures along the ![]() axis in the symmetry plane defined by the
axis in the symmetry plane defined by the ![]() axis (Figure 3b). For example, in plane [
axis (Figure 3b). For example, in plane [![]() ,
,![]() ], we consider
], we consider ![]() and
and ![]() because we can find
because we can find ![]() either by fitting along
either by fitting along ![]() or
or ![]() axis with
axis with ![]() as the axis defining the symmetry plane. The expressions for the anelliptic parameters are as follows:
as the axis defining the symmetry plane. The expressions for the anelliptic parameters are as follows:
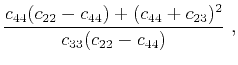 |
(5) | ||
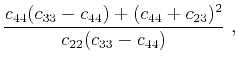 |
(6) | ||
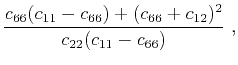 |
(7) | ||
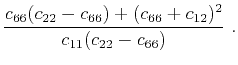 |
(8) |
| Schemes | Parameters | Acoustic Approx. | |
|
|
|||
|
|
|||
|
|
|||
|
|
|||
|
|
|||
On the basis of the Muir-Dellinger parameters in TI and orthorhombic media introduced in this section, we propose novel phase- and group-velocity approximations for qP waves in the subsequent sections. We first suggest a symmetric extension of the velocity approximations in TI media by Fomel (2004) and also extend the expressions to the orthorhombic case. We then utilize the relationships from Figure 1 to reduce the number of independent parameters in the proposed approximations to three and six in TI and orthorhombic media respectively so that they require the similar number of parameters as the other previously suggested approximations.
|
|
|
|
On anelliptic approximations for qP velocities in TI and orthorhombic media |