Non-hyperbolic common reflection surface

Apendix A: Hyperbolic reflector

In this appendix, we reproduce the derivation of an analytical expression for reflection traveltime from a hyperbolic reflector in a homogeneous velocity model (Fomel and Stovas, 2010). Similar derivations apply to an elliptic reflector and were used previously in the theory of offset continuation (Stovas and Fomel, 1996; Fomel, 2003).

Consider the source point $s$ and the receiver point $r$ at the surface $z=0$ above a 2-D constant-velocity medium and a hyperbolic reflector defined by the equation

$$z(x) = \sqrt{z_0^2 + x^2\,\tan^2{\alpha}}\;.$$ (19)

The reflection traveltime as a function of the reflection point location $y$ is

$$t = \frac{\sqrt{(s-y)^2 + z^2(y)} + \sqrt{(r-y)^2+z^2(y)}}{V}\;.$$ (20)

According to Fermat's principle, the traveltime should be stationary with respect to the reflection point $y$ :

$$0 = \frac{\partial T}{\partial y} = \frac{y-s + y\,\tan^2{\alpha}}{V\,\sqrt{(s-y)^2 + z_0^2 + y^2\,\tan^2{\alpha}}}$$

$$+ \frac{y-r + y\,\tan^2{\alpha}}{V\,\sqrt{(r-y)^2 + z_0^2 + y^2\,\tan^2{\alpha}}}\;.$$ (21)

Putting two terms in equation (A-3) on the different sides of the equation, squaring them, and reducing their difference to a common denominator, we arrive at the following quadratic equation with respect to $y$ :

$$y^2\,(s+r)\,\tan^2{\alpha} - 2\,y\,\left(s\,r\,\sin^2{\alpha} - z_0^2\right)$$

$$- z_0^2\,(s+r)\,\cos^2{\alpha} = 0\;.$$ (22)

Only one of the two branches of the solution

$$y = \frac{z_0^2\,(s+r)\,\cos^2{\alpha}}{z_0^2 - s\,r\,\sin^2{\alpha} + \sqrt{(z_0^2+s^2\,\sin^2{\alpha})\,(z_0^2+r^2\,\sin^2{\alpha})}}$$

has physical meaning. Substituting this solution into equation (A-2), we obtain, after a number of algebraic simplifications,

$$t^2 = \frac{2 z_0^2 + s^2 + r^2 - 2\,s\,r\,\cos^2{\alpha}}{V^2}$$

$$+ \frac{2\,\sqrt{(z_0^2+s^2\,\sin^2{\alpha})\,(z_0^2+r^2\,\sin^2{\alpha})}}{V^2}\;.$$ (23)

Making the variable change in equation (A-5) from $s$ and $r$ to midpoint and half-offset coordinates $m$ and $h$ according to $s=m-h=m_0+d-h$ , $r=m+h=m_0+d+h$ , we transform this equation to form (12), where the following correspondence between parameters is applied:

$$z_0^2 = \frac{t_0^2\,a_2}{(a_1^2+a_2)\,(a_1^2+b_2)}\;,$$ (24)

$$m_0 = \frac{t_0\,a_1}{a_1^2+a_2}\;,$$ (25)

$$\sin^2{\alpha} = \frac{a_1^2 + a_2}{a_1^2 + b_2}\;,$$ (26)

$$V^2 = \frac{4}{a_1^2 + b_2}\;.$$ (27)

The inverse relationships are given by

$$t_0 = \frac{2\,\sqrt{m_0^2\,\sin^2{\alpha} + z_0^2}}{V}\;,$$ (28)

$$a_1 = \frac{2\,m_0\,\sin^2{\alpha}}{V\,\sqrt{m_0^2\,\sin^2{\alpha} + z_0^2}}\;,$$ (29)

$$a_2 = \frac{4\,z_0^2\,\sin^2{\alpha}}{V^2\,\left(m_0^2\,\sin^2{\alpha} + z_0^2\right)}\;,$$ (30)

$$b_2 = \frac{4\,\left(m_0^2\,\sin^2{\alpha}\,\cos^2{\alpha}+z_0^2\right)}{V^2\,\left(m_0^2\,\sin^2{\alpha} + z_0^2\right)}\;.$$ (31)

The connection with the multifocusing parameters is summarized in Table 1 for the general case and three special cases (a plane dipping reflector, a flat reflector, and a point diffractor). The first two special cases turn the nonhyperbolic CRS equation into the hyperbolic form (8). The last case turns it into the double-square-root form (13).

	$t_0$	$K_{NIP}$	$K_N$	$\sin{\beta}$
Hyperbolic reflector	$\frac{2\,\sqrt{m_0^2\,\sin^2{\alpha} + z_0^2}}{V}$	$\frac{1}{\sqrt{m_0^2\,\sin^2{\alpha} + z_0^2}}$	$K_{NIP}\,\frac{z_0^2\,\sin^2{\alpha}}{m_0^2\,\sin^2{\alpha}\,\cos^2{\alpha}+z_0^2}$	$\frac{m_0\,\sin^2{\alpha}}{\sqrt{m_0^2\,\sin^2{\alpha} + z_0^2}}$
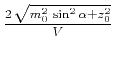
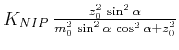
Plane dipping reflector
$z_0=0$	$\frac{2\,m_0\,\sin{\alpha}}{V}$	$\frac{1}{m_0\,\sin{\alpha}}$	0	$\sin{\alpha}$
Flat reflector
$\alpha=0$	$\frac{2\,z_0}{V}$	$\frac{1}{z_0}$	0	0
Point diffractor
$\alpha=\pi/2$	$\frac{2\,\sqrt{m_0^2 + z_0^2}}{V}$	$\frac{1}{\sqrt{m_0^2 + z_0^2}}$	$K_{NIP}$	$\frac{m_0}{\sqrt{m_0^2 + z_0^2}}$

Table 1. Multifocusing parameters for a hyperbolic reflector in a homogeneous medium ($V_0=V$ ). The general case and three special cases.

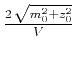
Non-hyperbolic common reflection surface