Theory of differential offset continuation

Integral offset continuation operator

Equation (1) describes a continuous process of reflected wavefield continuation in the time-offset-midpoint domain. In order to find an integral-type operator that performs the one-step offset continuation, I consider the following initial-value problem for equation (1):

Given a post-NMO constant-offset section at half-offset $h_1$

$$\left.P(t_n,h,y)\right\vert _{h=h_1}=P^{(0)}_1(t_n,y)$$ (62)

and its first-order derivative with respect to offset

$$\left.\partial P(t_n,h,y)\over \partial h\right\vert _{h=h_1}=P^{(1)}_1(t_n,y)\;,$$ (63)

find the corresponding section $P^{(0)}(t_n,y)$ at offset $h$.

Equation (1) belongs to the hyperbolic type, with the offset coordinate $h$ being a ``time-like'' variable and the midpoint coordinate $y$ and the time $t_n$ being ``space-like'' variables. The last condition (63) is required for the initial value problem to be well-posed (Courant, 1962). From a physical point of view, its role is to separate the two different wave-like processes embedded in equation (1), which are analogous to inward and outward wave propagation. We will associate the first process with continuation to a larger offset and the second one with continuation to a smaller offset. Though the offset derivatives of data are not measured in practice, they can be estimated from the data at neighboring offsets by a finite-difference approximation. Selecting a propagation branch explicitly, for example by considering the high-frequency asymptotics of the continuation operators, can allow us to eliminate the need for condition (63). In this section, I discuss the exact integral solution of the OC equation and analyze its asymptotics.

The integral solution of problem (62-63) for equation (1) is obtained in with the help of the classic methods of mathematical physics (Fomel, 2001,1994). It takes the explicit form

$$P(t_n,h,y) = \int\!\!\int P^{(0)}_1(t_1,y_1)\,G_0(t_1,h_1,y_1;t_n,h,y)\,dt_1\,dy_1$$

$$+ \int\!\!\int P^{(1)}_1(t_1,y_1)\,G_1(t_1,h_1,y_1;t_n,h,y)\,dt_1\,dy_1\;,$$ (64)

where the Green's functions $G_0$ and $G_1$ are expressed as

$$G_0(t_1,h_1,y_1;t_n,h,y) = \mbox{sign}(h-h_1)\,{H(t_n) \over \pi}\, {\partial \over \partial t_n}\,\left\{ H(\Theta) \over \sqrt{\Theta}\right\}\;,$$ (65)

$$G_1(t_1,h_1,y_1;t_n,h,y) = \mbox{sign}(h-h_1)\, {H(t_n) \over \pi}\,h\, {t_n \over t_1^2}\,\left\{ H(\Theta) \over \sqrt{\Theta}\right\}\;,$$ (66)

and the parameter $\Theta$ is

$$\Theta(t_1,h_1,y_1;t_n,h,y) = \left(h_1^2/t_1^2-h^2/t_n^2\right)\,\left(t_1^2-t_n^2\right)- \left(y_1-y\right)^2\;.$$ (67)

$H$ stands for the Heaviside step-function.

From equations (65) and (66) one can see that the impulse response of the offset continuation operator is discontinuous in the time-offset-midpoint space on a surface defined by the equality

$$\Theta(t_1,h_1,y_1;t_n,h,y) = 0\;,$$ (68)

which describes the ``wavefronts'' of the offset continuation process. In terms of the theory of characteristics (Courant, 1962), the surface $\Theta=0$ corresponds to the characteristic conoid formed by the bi-characteristics of equation (1) - time rays emerging from the point $\{t_n,h,y\}=\{t_1,h_1,y_1\}$. The common-offset slices of the characteristic conoid are shown in the left plot of Figure 5.

cont
Figure 5. Constant-offset sections of the characteristic conoid - ``offset continuation fronts'' (left), and branches of the conoid used in the integral OC operator (right). The upper part of the plots (small times) corresponds to continuation to smaller offsets; the lower part (large times) corresponds to larger offsets.

As a second-order differential equation of the hyperbolic type, equation (1) describes two different processes. The first process is ``forward'' continuation from smaller to larger offsets, the second one is ``reverse'' continuation in the opposite direction. These two processes are clearly separated in the high-frequency asymptotics of operator (64). To obtain the asymptotic representation, it is sufficient to note that ${1 \over \sqrt{\pi}}\, {H(t) \over \sqrt{t}}$ is the impulse response of the causal half-order integration operator and that $H(t^2-a^2) \over \sqrt{t^2-a^2}$ is asymptotically equivalent to $H(t-a) \over {\sqrt{2a}\,\sqrt{t-a}}$ $(t, a >0)$. Thus, the asymptotical form of the integral offset-continuation operator becomes

$$P^{(\pm)}(t_n,h,y) = {\bf D}^{1/2}_{\pm\,t_n}\,\int w^{(\pm)}_0(\xi;h_1,h,t_n)\, P^{(0)}_1(\theta^{(\pm)}(\xi;h_1,h,t_n),y_1-\xi)\,d\xi$$

$$\pm {\bf I}^{1/2}_{\pm\,t_n}\,\int w^{(\pm)}_1(\xi;h_1,h,t_n)\, P^{(1)}_1(\theta^{(\pm)}(\xi;h_1,h,t_n),y_1-\xi)\,d\xi\;.$$ (69)

Here the signs ``$+$'' and ``$-$'' correspond to the type of continuation (the sign of ${h-h_1}$), ${\bf D}^{1/2}_{\pm\,t_n}$ and ${\bf I}^{1/2}_{\pm\,t_n}$ stand for the operators of causal and anticausal half-order differentiation and integration applied with respect to the time variable $t_n$, the summation paths $\theta^{(\pm)}(\xi;h_1,h,t_n)$ correspond to the two non-negative sections of the characteristic conoid (68) (Figure 5):

$$t_1=\theta^{(\pm)}(\xi;h_1,h,t_n)= {t_n \over h}\,\sqrt{{U \pm V} \over 2 }\;,$$ (70)

where $U=h^2+h_1^2-\xi^2$, and $V=\sqrt{U^2-4\,h^2\,h_1^2}$; $\xi$ is the midpoint separation (the integration parameter), and $w^{(\pm)}_0$ and $w^{(\pm)}_1$ are the following weighting functions:

$$w^{(\pm)}_0 = {1 \over \sqrt{2\,\pi}}\, {\theta^{(\pm)}(\xi;h_1,h,t_n) \over \sqrt{t_n\,V}}\;,$$ (71)

$$w^{(\pm)}_1 = {1 \over \sqrt{2\,\pi}}\, {{\sqrt{t_n}\, h_1} \over {\sqrt{V}\,\theta^{(\pm)}(\xi;h_1,h,t_n)}}\;.$$ (72)

Expression (70) for the summation path of the OC operator was obtained previously by Stovas and Fomel (1996) and Biondi and Chemingui (1994). A somewhat different form of it is proposed by Bagaini and Spagnolini (1996). I describe the kinematic interpretation of formula (70) in Appendix B.

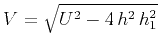In the high-frequency asymptotics, it is possible to replace the two terms in equation (69) with a single term (Fomel, 2003a). The single-term expression is

$$P^{(\pm)}(t_n,h,y) = {\bf D}^{1/2}_{\pm\,t_n}\,\int w^{(\pm... ...n)\, P^{(0)}_1(\theta^{(\pm)}(\xi;h_1,h,t_n),y_1-\xi)\,d\xi\;,$$ (73)

where

$$w^{(+)} = \sqrt{\theta^{(+)}(\xi;h_1,h,t_n) \over {2\,\pi}}\; {{h^2-h_1^2-\xi^2} \over {V^{3/2}}}\;,$$ (74)

$$w^{(-)} = {{\theta^{(-)}(\xi;h_1,h,t_n)} \over \sqrt{2\,\pi t_n}}\; {{h_1^2-h^2 +\xi^2} \over {V^{3/2}}}\;.$$ (75)

A more general approach to true-amplitude asymptotic offset continuation is developed by ().

The limit of expression (70) for the output offset $h$ approaching zero can be evaluated by L'Hospitale's rule. As one would expect, it coincides with the well-known expression for the summation path of the integral DMO operator (Deregowski and Rocca, 1981)

$$t_1=\theta^{(-)}(\xi;h_1,0,t_n)= \lim_{h \rightarrow 0} {{t_... ...t{{U - V} \over 2 }}= {{t_n\,h_1} \over \sqrt{h_1^2-\xi^2}}\;.$$ (76)

I discuss the connection between offset continuation and DMO in the next section.

Theory of differential offset continuation