Angle-gather time migration

Traveltime considerations

rays Figure 1. Reflection rays in a constant-velocity medium: a scheme.

Let us consider a simple reflection experiment in an effectively constant-velocity medium, as depicted in Figure 1. The pair of incident and reflected rays and the line between the source $s$ and the receiver $r$ form a triangle in space. From the trigonometry of that triangle we can derive simple relationships among all the variables of the experiment (Fomel, 1995,1996a,1997).

Introducing the dip angle $\alpha$ and the reflection angle $\gamma$, the total reflection traveltime $t$ can be expressed from the law of sines as

$$t = {\frac{2 h}{v}} {\frac{\cos(\alpha+\gamma) + \cos(\a... ...ma}}} = {\frac{2 h}{v} \frac{\cos{\alpha}}{\sin{\gamma}}}\;,$$ (1)

where $v$ is the medium velocity, and $h$ is the half-offset between the source and the receiver.

Additionally, by following simple trigonometry, we can connect the half-offset $h$ with the depth of the reflection point $z$, as follows:

$$h = {\frac{z}{2}} {\frac{\sin{2 \gamma}}{2 \cos(\alpha... ...in{\gamma} \cos{\gamma}}{\cos^2{\alpha} - \sin^2{\gamma}}}\;.$$ (2)

Finally, the horizontal distance between the midpoint $x$ and the reflection point $\xi$ is

$$x - \xi = h \frac{\cos(\alpha-\gamma) \sin(\alpha+\gamm... ...,\frac{\sin{\alpha} \cos{\alpha}}{\sin{\gamma} \cos{\gamma}}$$ (3)

Equations (1-3) completely define the kinematics of angle-gather migration. Regrouping the terms, we can rewrite the three equations in a more symmetric form:

$$t = \frac{2 z}{v} \frac{\cos{\alpha} \cos{\gamma}}{\cos^2{\alpha} - \sin^2{\gamma}}$$ (4)

$$h = z \frac{\sin{\gamma} \cos{\gamma}}{\cos^2{\alpha} - \sin^2{\gamma}}$$ (5)

$$x - \xi = z \frac{\sin{\alpha} \cos{\alpha}}{\cos^2{\alpha} - \sin^2{\gamma}}$$ (6)

For completeness, here is the inverse transformation from $t$, $h$, and $x-\xi$ to $z$, $\gamma$, and $\alpha$:

$$z^2 = \frac{ \left[(v t/2)^2 - (x-\xi)^2\right] \left[(v t/2)^2 - h^2\right] }{(v t/2)^2}$$ (7)

$$\sin^2{\gamma} = \frac{h^2 \left[(v t/2)^2 - (x-\xi)^2\right]} {(v t/2)^4 - h^2 (x-\xi)^2}$$ (8)

$$\cos^2{\alpha} = \frac{(v t/2)^2 \left[(v t/2)^2 - (x-\xi)^2\right]} {(v t/2)^4 - h^2 (x-\xi)^2}$$ (9)

The inverse transformation (7-9) can be found by formally solving system (4-6).

The lines of constant reflection angle $\gamma$ and variable dip angle $\alpha$ for a given position of a reflection (diffraction) point $\{z,\xi\}$ have the meaning of summation curves for angle-gather Kirchhoff migration. The whole range of such curves for all possible values of $\gamma$ covers the diffraction traveltime surface - ``Cheops' pyramid'' (Claerbout, 1985) in the $\{t,x,h\}$ space of seismic reflection data. As pointed out by Fowler (1997), this condition is sufficient for proving the kinematic validity of the angle-gather approach. For comparison, Figure 2 shows the diffraction traveltime pyramid from a diffractor at 0.5 km depth. The pyramid is composed of common-offset summation curves of the conventional time migration. Figure 3 shows the same pyramid composed of constant-$\gamma$ curves of the angle-gather migration.

coffset Figure 2. Traveltime pyramid, composed of common-offset summation curves.

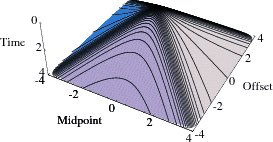
cangle Figure 3. Traveltime pyramid, composed of common-reflection-angle summation curves.

The most straightforward Kirchhoff algorithm of angle-gather migration can be formulated as follows:

• For each reflection angle $\gamma$ and each dip angle $\alpha$,
  • For each output location $\{z,\xi\}$,
    1. Find the traveltime $t$, half-offset $h$, and midpoint $x$ from formulas (4), (5), and (6) respectively.
    2. Stack the input data values into the output.

As follows from equations (4-6), the range of possible $\alpha$'s should satisfy the condition

$$\cos^2{\alpha} > \sin^2{\gamma}\quad\mbox{or}\quad \vert\alpha\vert + \vert\gamma\vert < \frac{\pi}{2}\;.$$ (10)

The described algorithm is not the most optimal in terms of the input/output organization, but it can serve as a basic implementation of the angle-gather idea. The stacking step requires an appropriate weighting. We discuss the weighting issues in the next section.

Angle-gather time migration