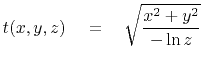|
 |
 |
 | Plane waves in three dimensions |  |
![[pdf]](icons/pdf.png) |
Next: 3-D SPECTRAL FACTORIZATION
Up: GRADIENT ALONG THE BEDDING
Previous: Definition of LOMOPLAN in
Figure 4
used a model called ``Sigmoid.''
Using the same modeling concepts,
I set out to make a three-dimensional model.
The model has horizontal layers near the top,
a Gaussian appearance in the middle, and dipping layers on the bottom,
with horizontal unconformities between the three regions.
Figure 8 shows
a vertical slice through the 3-D ``qdome'' model
and components of its LOMOPLAN.
There is also a fault that will be described later.

|
|---|
qdomesico
Figure 8.
Left is a vertical slice through the 3-D ``qdome'' model.
Center is the in-line component of the LOMOPLAN.
Right is the cross-line component of the LOMOPLAN.
|
|---|
![[pdf]](icons/pdf.png) ![[png]](icons/viewmag.png) ![[scons]](icons/configure.png)
|
|---|
The most interesting part of the qdome model is the Gaussian center.
I started from the equation of a Gaussian
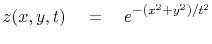 |
(3) |
and backsolved for 
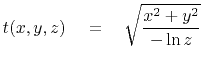 |
(4) |
Then I used a random-number generator
to make a blocky one-dimensional impedance function of  .
At each
.
At each 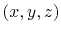 location in the model
I used the impedance at time
location in the model
I used the impedance at time 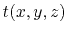 ,
and finally defined reflectivity as the logarithmic derivative
of the impedance.
Without careful interpolation (particularly where the beds pinch out)
a variety of curious artifacts appear.
I hope to find time to
use the experience of making the qdome model
to make a tutorial lesson on interpolation.
A refinement to the model is that within a certain subvolume
the time
,
and finally defined reflectivity as the logarithmic derivative
of the impedance.
Without careful interpolation (particularly where the beds pinch out)
a variety of curious artifacts appear.
I hope to find time to
use the experience of making the qdome model
to make a tutorial lesson on interpolation.
A refinement to the model is that within a certain subvolume
the time 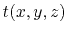 is given a small additive constant.
This gives a fault along the edge of the subvolume.
Ray Abma defined the subvolume for me in the qdome model.
The fault looks quite realistic,
and it is easy to make faults of any shape,
though I wonder how they would relate to realistic fault dynamics.
Figure 9 shows
a top view of
the 3-D qdome model
and components of its LOMOPLAN.
Notice that the cross-line spacing
has been chosen to be double the in-line spacing.
Evidently a consequence of this,
in both
Figure 8 and
Figure 9, is that the Gaussian dome is not so well suppressed
on the crossline cut as on the in-line cut.
By comparison, notice that the horizontal bedding above the dome
is perfectly suppressed,
whereas the dipping bedding below the dome is imperfectly suppressed.
is given a small additive constant.
This gives a fault along the edge of the subvolume.
Ray Abma defined the subvolume for me in the qdome model.
The fault looks quite realistic,
and it is easy to make faults of any shape,
though I wonder how they would relate to realistic fault dynamics.
Figure 9 shows
a top view of
the 3-D qdome model
and components of its LOMOPLAN.
Notice that the cross-line spacing
has been chosen to be double the in-line spacing.
Evidently a consequence of this,
in both
Figure 8 and
Figure 9, is that the Gaussian dome is not so well suppressed
on the crossline cut as on the in-line cut.
By comparison, notice that the horizontal bedding above the dome
is perfectly suppressed,
whereas the dipping bedding below the dome is imperfectly suppressed.

|
|---|
qdometoco
Figure 9.
Left is a horizontal slice through the 3-D qdome model.
Center is the in-line component of the LOMOPLAN.
Right is the cross-line component of the LOMOPLAN.
|
|---|
![[pdf]](icons/pdf.png) ![[png]](icons/viewmag.png) ![[scons]](icons/configure.png)
|
|---|
Finally, I became irritated at the need to look at two output volumes.
Because I rarely if ever interpreted the polarity of the LOMOPLAN components,
I formed their sum of squares and show the single
square root volume in Figure 10.

|
|---|
qdometora
Figure 10.
Left is the model.
Right is the magnitude of the LOMOPLAN
components in Figure 9.
|
|---|
![[pdf]](icons/pdf.png) ![[png]](icons/viewmag.png) ![[scons]](icons/configure.png)
|
|---|
 |
 |
 |
 | Plane waves in three dimensions |  |
![[pdf]](icons/pdf.png) |
Next: 3-D SPECTRAL FACTORIZATION
Up: GRADIENT ALONG THE BEDDING
Previous: Definition of LOMOPLAN in
2013-07-27