Selecting an optimal aperture in Kirchhoff migration using dip-angle images

Appendix: Hyperbolic reflector in the dip-angle domain

For insight into the appearance of reflector images in the dip-angle domain, let us consider the case of a hyperbolic reflector (Fomel and Kazinnik, 2013). A special property of hyperbolic reflectors is that they can transform to plane dipping reflectors or point diffractors with an appropriate choice of parameters. Reflector depth is given by the function

$$z(x)=\sqrt{z_0^2+(x-x_0)^2 \tan^2\beta}\;,$$ (11)

and zero-offset reflection traveltime is given by

$$t(y)=\frac{2}{v} \sqrt{z_0^2+(y-x_0)^2 \sin^2\beta}\;.$$ (12)

When the reflector is imaged by time migration in the dip-angle domain (Sava and Fomel, 2003) using velocity $v_m$, point $\{y,t\}$ in the data domain migrates to $\{x_m,t_m\}$ in the image domain according to

$$x_m = y - \frac{v_m}{2} t \sin{\alpha} = \displaystyle y - \frac{v_m \sin{\alpha}}{v} \sqrt{z_0^2+(y-x_0)^2 \sin^2\beta}\;,$$ (13)

$$t_m = t \cos{\alpha} = \displaystyle \frac{2 \cos{\alpha}}{v} \sqrt{z_0^2+(y-x_0)^2 \sin^2\beta}\;,$$ (14)

where $\alpha$ is the migration dip angle. Eliminating $y$ from equations A-3 and A-4, we arrive at the equation

$$t_m(x_m) = \frac{2 \cos{\alpha}}{v} \frac{\gamma (x_m-x_... ...n^2{\beta} + \sqrt{(x_m-x_0)^2 \sin^2{\beta}+z_0^2 D}}{D}\;,$$ (15)

where $\gamma=v_m/v$ and $D=1-\gamma^2 \sin^2{\alpha} \sin^2{\beta}$. Equation A-5 describes the shape of the image of the hyperbolic reflector (A-1) in the dip-angle domain.

When the dip of the migrated event, imaged at a correct velocity ($\gamma=1$),

$$\tan{\alpha_m} = \displaystyle \frac{v}{2} t_m'(x_m) = \... ...m-x_0}{\sqrt{(x_m-x_0)^2 \sin^2{\beta}+z_0^2 D}}\right]\;,$$ (16)

is equal to the dip of the image ( $\alpha_m=\alpha$), it also becomes equal to the true dip of the reflector ( $\alpha_m=\alpha_0$), where

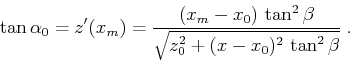$$\tan{\alpha_0} = z'(x_m) = \frac{(x_m-x_0) \tan^2{\beta}}{\sqrt{z_0^2+(x-x_0)^2 \tan^2\beta}}\;.$$ (17)

We can specify these conditions for two special cases described next.

Selecting an optimal aperture in Kirchhoff migration using dip-angle images