Next: Snell waves
Up: DIPPING WAVES
Previous: DIPPING WAVES
It is natural to begin studies of waves
with equations that describe plane waves
in a medium of constant velocity.
Figure 3.7 depicts a ray moving down into the earth
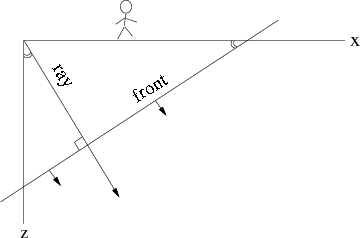
at an angle 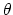 from the vertical.
from the vertical.
front
Figure 7.
Downgoing ray and wavefront.
|
|
![[pdf]](icons/pdf.png) ![[png]](icons/viewmag.png) ![[xfig]](icons/xfig.png)
|
|---|
Perpendicular to the ray is a wavefront.
By elementary geometry the angle between the wavefront
and the earth's surface
is also 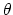 .
The ray increases its length at a speed
.
The ray increases its length at a speed 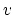 .
The speed that is observable on the earth's surface is the intercept
of the wavefront with the earth's surface.
This speed, namely
.
The speed that is observable on the earth's surface is the intercept
of the wavefront with the earth's surface.
This speed, namely
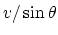 , is faster than
, is faster than 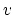 .
Likewise, the speed of the intercept of the wavefront and
the vertical axis is
.
Likewise, the speed of the intercept of the wavefront and
the vertical axis is
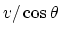 .
A mathematical expression for a straight line
like that shown to be the wavefront in Figure 3.7 is
.
A mathematical expression for a straight line
like that shown to be the wavefront in Figure 3.7 is
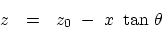 |
(4) |
In this expression 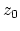 is the intercept between the wavefront
and the vertical axis.
To make the intercept move downward, replace it by the
appropriate velocity times time:
is the intercept between the wavefront
and the vertical axis.
To make the intercept move downward, replace it by the
appropriate velocity times time:
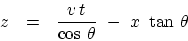 |
(5) |
Solving for time gives
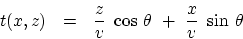 |
(6) |
Equation (3.6) tells the time that the wavefront will pass any
particular location 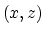 .
The expression for a shifted waveform
of arbitrary shape is
.
The expression for a shifted waveform
of arbitrary shape is 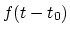 .
Using (3.6) to define the time shift
.
Using (3.6) to define the time shift 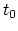 gives an expression for
a wavefield that is some waveform moving on a ray.
gives an expression for
a wavefield that is some waveform moving on a ray.
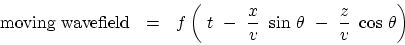 |
(7) |
Next: Snell waves
Up: DIPPING WAVES
Previous: DIPPING WAVES
2009-03-16
![]() from the vertical.
from the vertical.

![]() is the intercept between the wavefront
and the vertical axis.
To make the intercept move downward, replace it by the
appropriate velocity times time:
is the intercept between the wavefront
and the vertical axis.
To make the intercept move downward, replace it by the
appropriate velocity times time: